Dr. Dawn's blog

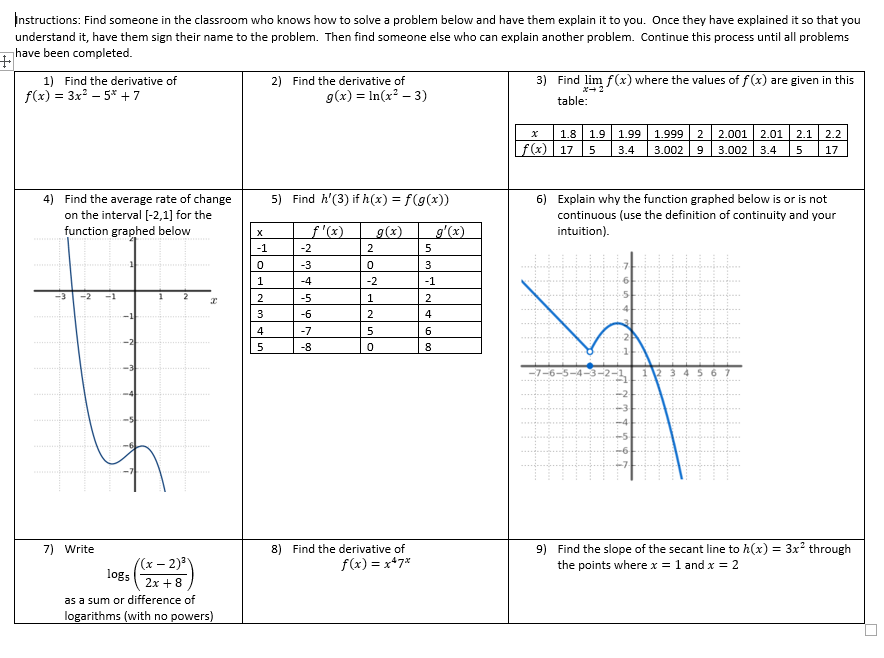
For the  review for test 1 in my Business Calculus course, I used the “Find Someone Who” activity featured in a previous post.  When it was time to review for the second test, the students asked if we would be doing that again.  Unfortunately, it didnt’ feel like a good fit with the content at that point in the class.  So instead I concocted a low budget escape room.  The pdf of my escape room is available here.  If you want the .tex code you can acces it here, but I took out the QR code and link to my form, since you’ll need your own.  If you want to try it our before you adapt it to your class, feel free to submit your answers to my Google Form (use the QR code in the pdf).

I got the idea for a digital escape room from a video I watched early in the pandemic.  I learned the details of how to set one up using Google Forms from Bespoke ELA ‘s blog post.  She explains the mechanics very well, so I won’t go into the details here.  However, I will mention that the key step is to use “response validation” in the Google Form so that the students can’t go on unless they have entered the correct code.

For me the hardest part was thinking of a suitable story to frame the escape room and finding the right “voice”/writing style.  In the end I chose to have the students need to find the manual over ride for the self-destruct sequence on our interstellar spaceship.  I wrote in a campy style reminiscent ot the Choose Your Own Adventure books of my youth.

Here’s the introduction to my escape room:

We are the crew of the intergalactic spacecraft the USS Titan*. Captain Archey tripped and fell. On her way down, she hit her head on the self destruct button**. Now, she is unconscious and the 50 minute self-destruct count down has begun. Without the captain’s voice print, the only way to deactivate the self-destruct sequence is using the manual override switch.

The communications officer says, “I remember Captain Archey mentioning the instructions to deactivate it being on her e-reader.”

The exobiologist says, “I bet her e-reader is in her quarters. Does anyone know the passcode to enter her quarters?”

The head of security says, “She told me she left a hint to the code on a paper in her shoe whenever she had to change the code. Let’s hope she changed it recently.”  The head of security removes Captain Archey’s shoe and finds the next page of this packet.

* Our university mascot is the Titans.

**I had three students at the front of the room to be the officers and read their parts.  At this point in the story I flopped down on the floor and stayed down throughout the introduction.

All the “locks” in the escape room were codes to enter in the Google Form until the very end.  After the students enter

ed the third code, which was the combination to my gym locker, they could head over to the front corner of the room where I had a laptop case/small carry on suitcase labled, “Captain Archey’s gym locker.”

Inside the “gym locker” they found a “key” (a picture of a key on cardstock) and a note indicating that the door to the manual shut off was in the back corner of the room.

In the back corner of the room there happened to be a little funny space between the wall and the window.  Inside that space I put a switch controlling a red light (made of my kids’ Snap Circuits) and some cheap prizes like Lifesavers and pencils.  To cover the space I had taped up a piece of paper with a door knob on it.

It was all kind of silly, but the students were good sports.  One even asked me, “Are we really going to die if we don’t finish all these problems?”

I was actually walking around the room, but because in the story I was unconcious, the students made more of an effort to figure things out on their own (for example, digging through their notes rather than asking me for a formula).

Two of the three groups of students finished the activity and the third group was close.  I was afraid that the students who didn’t finish might feel discouraged or disaapointed, but they actually came up to me after class and said what a great activity it was.

It’s a perennial problem in math classes: Its review day for the test and the professor asks “are there any questions?” only to be met with silence.

Of course there are many reasons students might not ask questions.  Probably the main ones are:

They haven’t started reviewing yet, so they don’t know what questions they have.

They have mostly mastered the material and don’t need to ask questions (this is probably a very small minority).

They aren’t comfortable to ask a question.

Of course there are many reasons why a student might not be comfortable enough to ask a question: thinking that asking is a sign of weakness, worry their their question is dumb, fear that they are dumb or others will think they are, because the professor is an authority figure, stereotype threat,  a past traumatic experience asking a question in a class, or difficulty formulating a coherent question to name a few.

Whatever the reason for the lack of questions, students need to ask questions in order to succeed mathematically.  Therefore, in an attempt to avoid the awkward staring at each other while the students don’t ask any questions, I decided to try a completely different review activity in my Business Calculus class this week.  The activity was called “Find someone who.”  I got the idea from the book Choosing to See: A Framework for Equity in the Math Classroom by Pamela Seda and Kyndall Brown.  They learned about it from the book Kagan Cooperative Learning.

In the “Find someone who” activity, the professor prepares a worksheet formatted like a bingo card/ tic-tac-toe board.  The students are asked to move around the room asking their classmates to explain a problem to them.  Once the student has understood how to solve the problem, the explainer signs that square on their worksheet.

This activity can be adapted for any topic.  Mine was the review for the test on the basics of derivatives (including limit intuition and secant lines).  You can access the PDF  here: FindSomeoneWhoTest1Review .  To tantalize you, I’ve also included a picture of the worksheet.

Handout for Find Someone Who

The energy in the room during this activity was fantastic!  At first most students only wanted to talk to the people next to them, but with some gentle prodding and some affirmations, the students got into it.  I got to hear fun snippets of conversation like one student explaining the chain rule to another, “and then you stuff it back in…”.

As a review for the test, this activity allowed students to solve problems (as most reviews do), but also allowed them to explain concepts and procedures which helps the explainer to learn and remember the material.

Besides serving as a review for the test, the activity served a few other functions.  Students got/had to ask questions in a non-threatening environment–everyone had to ask questions, so there was no stigma attached to asking.  Hopefully, this will also have a longer lasting effect of normalizing asking for help and increasing student willingness to ask questions of me and each other.  On a related note, the activity helps to “include others as experts” which is one of the pillar’s of Seda and Brown’s titular framework for equity. As the name suggests, this pillar is all about establishing that the teacher is not the one and only source of knowledge–other students and the student’s own self also bring valuable knowledge.

At the end of the class period, I asked students to write me a couple of sentences about how they thought the activity went.  I tried to encourage honest responses by being completely honest with them. I said, “I’ve never done an activity like this before; I read it in a book, so I want to know how it worked from your perspective.”  Most students were enthusiastic in their positive review of the activity.  The students also identified many benefits of the activity, such as:

Getting to know classmates

Teaching classmates helped me remember the steps

Greater confidence for the upcoming test

Hearing the steps out loud and in different ways

Experiencing the value of learning from peers

Awareness of weak spots in knowledge of the material, which will help with studying

I was delighted that the students were extremely engaged and that they were able to articulate some of the many benefits of the activity for themselves. This activity powerfully demonstrated that our classroom is a safe space for asking questions.

Sources:

Pamela Seda and Kyndall Brown, Choosing to See: A Framework for Equity in the Math Classroom (San Diego, CA: Dave Burgess Consulting Inc., 2021) https://www.daveburgessconsulting.com/books/choosing-to-see/

Spencer Kagan and Miguel Kagan, Kagan Cooperative Learning (San Clemente, CA: Kagan Publishing, 2009)  https://www.kaganonline.com/catalog/cooperative_learning.php#BKCLW

Here are two problems I used in my calculus 1 class, just after teaching them the definition of the derivative and discussing average and instantaneous rates of change.

1. My car has a digital read out with the average fuel economy.  I used to have a car which also had the instantaneous fuel economy.
   
   What is the difference between these two fuel economies?  Under what circumstances is each more useful?  How can I use the average fuel economy feature to approximate my instantaneous fuel economy?  (Your answer should be  2-5 complete sentences).
2. Read the article at
   http://www.slate.com/blogs/bad_astronomy/2015/09/03/ice_loss_greenland_and_antarctica_lost_5_trillion_tons_since_1992.html .
   You can use this QR code to access it
   
   1. What is the average rate of change of the mass of Antarctica’s ice?
   2. What is the average rate of change of the mass of Greenland’s ice?
   3. Looking at the graphs, do you think it is reasonable to talk about the instantaneous rate of change for these quantities?  Why or why not?
      Then answer the following questions. Give all answers in complete sentences with units.

The FoxTrot Strip from November 6, 2005 contained a “numerical word search”

You can find the strip here.

There are seven clues which are arithmetic problems, but there is also the derivative of 43981 x , the definite integral of x^3 from 0 to 16, and the sum from k equals 0 to 47 of k squared.    I asked my calculus students to perform all these calculations.